9 1b Ws Graphing Quadratic Functions Again

9.7: Graph Quadratic Functions Using Backdrop

1. Last updated

2. Save equally PDF

Page ID

18845

Learning Objectives

By the end of this section, y'all will exist able to:

• Recognize the graph of a quadratic function
• Notice the centrality of symmetry and vertex of a parabola
• Find the intercepts of a parabola
• Graph quadratic functions using backdrop
• Solve maximum and minimum applications

Before you get started, take this readiness quiz.

1. Graph the function \(f(x)=x^{2}\) by plotting points.
   If you lot missed this problem, review Case 3.54.
2. Solve: \(two x^{two}+3 x-2=0\).
   If you missed this problem, review Case 6.45.
3. Evaluate \(-\frac{b}{2 a}\) when \(a=3\) and \(b=-6\).
   If you lot missed this trouble, review Case 1.21.

Recognize the Graph of a Quadratic Function

Previously we very briefly looked at the role \(f(x)=x^{2}\), which we called the square role. Information technology was one of the first non-linear functions we looked at. Now we will graph functions of the course \(f(x)=a x^{two}+b x+c\) if \(a \neq 0\). Nosotros phone call this kind of office a quadratic function.

Definition \(\PageIndex{1}\)

A quadratic function, where \(a, b\), and \(c\) are real numbers and \(a≠0\), is a office of the grade

\(f(x)=a x^{2}+b 10+c\)

We graphed the quadratic function \(f(x)=10^{2}\) by plotting points.

Effigy 9.six.1

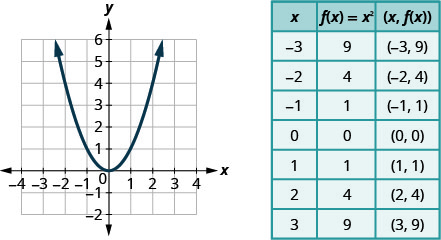
Every quadratic office has a graph that looks similar this. We call this figure a parabola. Let's practice graphing a parabola by plotting a few points.

Example \(\PageIndex{1}\)

Graph: \(f(x)=x^{2}-1\).

Solution:

Nosotros will graph the function by plotting points.

Choose integer values for \(x\), substitute them into the equation and simplify to find \(f(x)\). Record the values of the ordered pairs in the chart.
Plot the points, and then connect them with a smooth curve. The outcome volition be the graph of the function \(f(ten)=x^{2}-i\).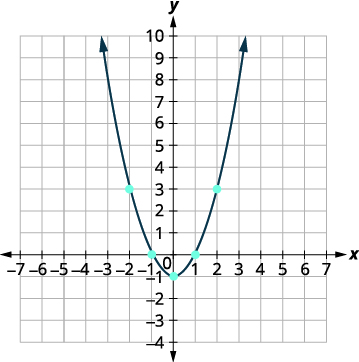

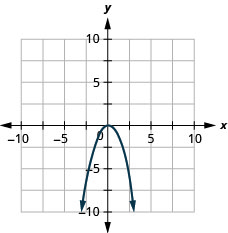
Exercise \(\PageIndex{1}\)

Graph \(f(x)=-10^{2}\).

Answer

Do \(\PageIndex{2}\)

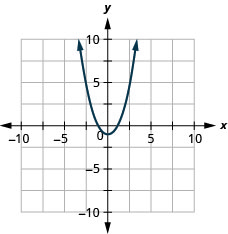
Graph \(f(x)=x^{2}+1\).

Answer

All graphs of quadratic functions of the course \(f(ten)=a x^{two}+b x+c\) are parabolas that open upward or downwards. See Figure ix.six.6

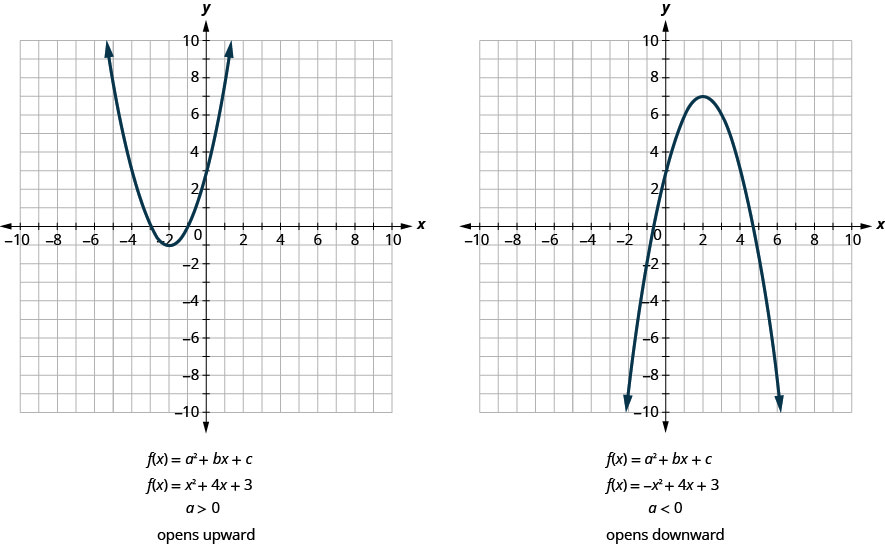
Detect that the only divergence in the two functions is the negative sign before the quadratic term (\(10^{ii}\) in the equation of the graph in Figure nine.half-dozen.vi). When the quadratic term, is positive, the parabola opens upward, and when the quadratic term is negative, the parabola opens down.

Definition \(\PageIndex{two}\)

Parabola Orientation

For the graph of the quadratic function \(f(10)=a x^{ii}+b x+c\), if

Example \(\PageIndex{two}\)

Determine whether each parabola opens upward or downward:

1. \(f(x)=-3 x^{2}+2 ten-four\)
2. \(f(ten)=6 x^{2}+seven x-nine\)

Solution:

a. Find the value of \(a\).

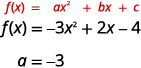
Since the \(a\) is negative, the parabola volition open downward.

b. Find the value of \(a\).

Since the \(a\) is positive, the parabola will open up upwards.

Exercise \(\PageIndex{3}\)

Determine whether the graph of each office is a parabola that opens up or downward:

1. \(f(ten)=two x^{two}+5 x-2\)
2. \(f(x)=-3 ten^{2}-4 x+seven\)

Answer

1. upwards
2. downward

Exercise \(\PageIndex{4}\)

Determine whether the graph of each role is a parabola that opens up or downward:

1. \(f(x)=-2 x^{ii}-2 ten-3\)
2. \(f(ten)=five x^{2}-2 x-one\)

Answer

1. down
2. upwardly

Discover the Axis of Symmetry and Vertex of a Parabola

Expect again at Figure ix.6.10. Do you see that we could fold each parabola in one-half and then ane side would lie on acme of the other? The 'fold line' is a line of symmetry. We call information technology the centrality of symmetry of the parabola.

We show the same two graphs again with the axis of symmetry.

The equation of the axis of symmetry can be derived past using the Quadratic Formula. Nosotros will omit the derivation hither and go on straight to using the result. The equation of the axis of symmetry of the graph of \(f(x)=a x^{2}+b x+c\) is \(x=-\frac{b}{2 a}\).

And so to find the equation of symmetry of each of the parabolas we graphed higher up, nosotros will substitute into the formula \(x=-\frac{b}{ii a}\).

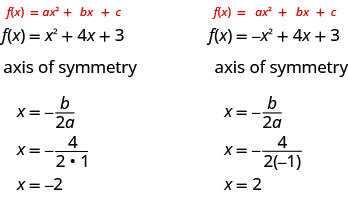
Detect that these are the equations of the dashed blue lines on the graphs.

The betoken on the parabola that is the everyman (parabola opens up), or the highest (parabola opens down), lies on the axis of symmetry. This point is called the vertex of the parabola.

We can hands discover the coordinates of the vertex, because we know information technology is on the axis of symmetry. This means its
\(10\)-coordinate is \(-\frac{b}{2 a}\). To notice the \(y\)-coordinate of the vertex we substitute the value of the \(10\)-coordinate into the quadratic function.

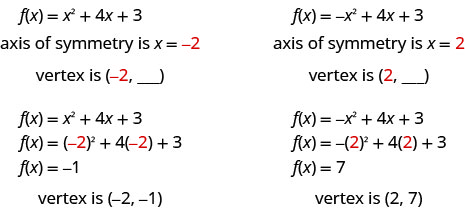
Centrality of Symmetry and Vertex of a Parabola

The graph of the function \(f(x)=a x^{2}+b x+c\) is a parabola where:

• the axis of symmetry is the vertical line \(10=-\frac{b}{2 a}\).
• the vertex is a point on the centrality of symmetry, and so its \(x\)-coordinate is \(-\frac{b}{2 a}\)
• the \(y\)-coordinate of the vertex is found past substituting \(x=-\frac{b}{2 a}\) into the quadratic equation.

Example \(\PageIndex{3}\)

For the graph of \(f(x)=3 x^{two}-6 10+2\) find:

1. the centrality of symmetry
2. the vertex

Solution:

a.

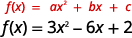The axis of symmetry is the vertical line \(x=-\frac{b}{ii a}\).
Substitute the values \(a,b\) into the equation.	\(ten=-\frac{-six}{2 \cdot 3}\)
Simplify.	\(10=1\)
	The axis of symmetry is the line \(x=1\).

b.

Do \(\PageIndex{5}\)

For the graph of \(f(x)=2 x^{ii}-eight x+1\) find:

1. the axis of symmetry
2. the vertex

Reply

1. \(x=2\)
2. \((2,-7)\)

Exercise \(\PageIndex{six}\)

For the graph of \(f(x)=2 ten^{two}-4 x-3\) find:

1. the axis of symmetry
2. the vertex

Respond

1. \(x=1\)
2. \((ane,-5)\)

Find the Intercepts of a Parabola

When we graphed linear equations, we frequently used the \(ten\)- and \(y\)-intercepts to assistance united states of america graph the lines. Finding the coordinates of the intercepts volition help the states to graph parabolas, too.

Remember, at the \(y\)-intercept the value of \(ten\) is nil. And then to observe the \(y\)-intercept, we substitute \(ten=0\) into the function.

Let's find the \(y\)-intercepts of the 2 parabolas shown in Figure 9.6.20.

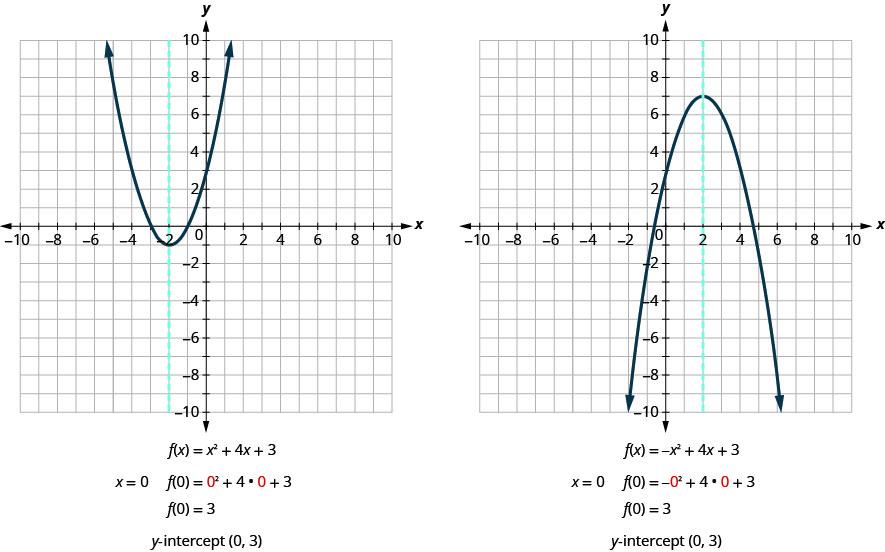
An \(ten\)-intercept results when the value of \(f(x)\) is cipher. To detect an \(x\)-intercept, nosotros allow \(f(10)=0\). In other words, we will need to solve the equation \(0=a x^{2}+b 10+c\) for \(x\).

\(\begin{aligned} f(10) &=a ten^{ii}+b x+c \\ 0 &=a x^{2}+b 10+c \end{aligned}\)

Solving quadratic equations similar this is exactly what nosotros have done earlier in this chapter!

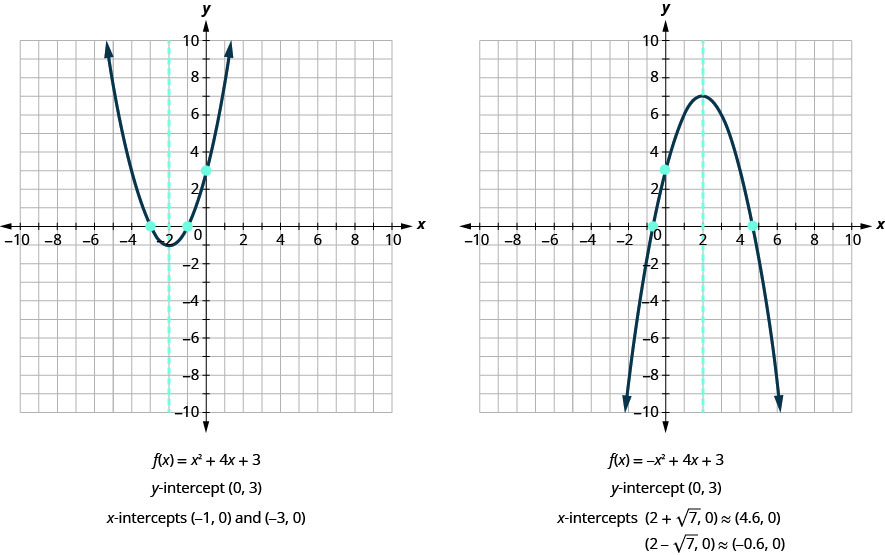
We tin now detect the \(x\)-intercepts of the two parabolas nosotros looked at. First we will find the \(x\)-intercepts of the parabola whose function is \(f(x)=ten^{ii}+4 x+iii\).

	\(f(x)=x^{two}+4 x+3\)
Let \(f(10)=0\).	\(\color{red}0\colour{blackness}=x^{2}+4 x+3\)
Gene.	\(0=(ten+1)(x+3)\)
Utilize the Zip Product Property.	\(x+i=0 \quad ten+3=0\)
Solve.	\(x=-1 \quad x=-3\)
	The \(x\)-intercepts are \((-1,0)\) and \((-iii,0)\).

Now nosotros will notice the \(x\)-intercepts of the parabola whose part is \(f(x)=-x^{2}+4 ten+3\).

	\(f(x)=-x^{2}+4 10+3\)
Let \(f(x)=0\).	\(\color{blood-red}0 \color{black}=-x^{2}+4 x+3\)
This quadratic does not gene, and so we use the Quadratic Formula.	\(x=\frac{-b \pm \sqrt{b^{two}-iv a c}}{2 a}\)
\(a=-1, b=iv, c=three\)	\(10=\frac{-four \pm \sqrt{4^{2}-iv(-one)(3)}}{2(-one)}\)
Simplify.	\(10=\frac{-four \pm \sqrt{28}}{-ii}\)
	\(x=\frac{-4 \pm 2 \sqrt{7}}{-two}\)
	\(x=\frac{-ii(2 \pm \sqrt{seven})}{-2}\)
	\(x=2 \pm \sqrt{vii}\)
	The \(ten\)-intercepts are \((2+\sqrt{7}, 0)\) and \((two-\sqrt{7}, 0)\).

We will use the decimal approximations of the \(x\)-intercepts, and then that we can locate these points on the graph,

\((2+\sqrt{7}, 0) \approx(4.6,0) \quad(2-\sqrt{seven}, 0) \approx(-0.half dozen,0)\)

Exercise these results agree with our graphs? Encounter Figure 9.6.34

Notice the Intercepts of a Parabola

To discover the intercepts of a parabola whose part is \(f(x)=a 10^{2}+b 10+c\):

\(y\)-intercept

Allow \(10=0\) and solve for \(f(x)\).

\(x\)-intercepts

Allow \(f(x)=0\) and solve for \(x\)

Instance \(\PageIndex{4}\)

Find the intercepts of the parabola whose part is \(f(x)=x^{two}-2 x-8\).

Solution:

To notice the \(y\)-intercept, let \(x=0\) and solve for \(f(x)\).	\(f(x)=10^{two}-two ten-8\)
	\(f(0)=\color{red}0\color{blackness}^{two}-2 \cdot \color{red}0 \color{black}-8\)
	\(f(0)=-8\)
	When \(ten=0\), then \(f(0)=-eight\). The \(y\)-intercept is the point \((0,-8)\).
To find the \(x\)-intercept, permit \(f(x)=0\) and solve for \(x\).	\(f(ten)=x^{ii}-2 x-eight\)
	\(0=ten^{2}-2 ten-8\)
Solve by factoring.	\(0=(x-four)(x+2)\)
	\(0=x-iv \quad 0=x+two\)
	\(4=x \quad-2=x\)
	When \(f(ten)=0\), and so \(x=four\) or \(x=-two\). The \(ten\)-intercepts are the points \((4,0)\) and \((-ii,0)\).

Exercise \(\PageIndex{seven}\)

Detect the intercepts of the parabola whose function is \(f(x)=x^{2}+2 x-eight\).

Answer

\(y\) -intercept: \((0,-8) x\) -intercepts \((-iv,0),(2,0)\)

Exercise \(\PageIndex{8}\)

Find the intercepts of the parabola whose part is \(f(x)=ten^{2}-iv 10-12\).

Answer

\(y\) -intercept: \((0,-12) ten\) -intercepts \((-2,0),(half dozen,0)\)

In this chapter, nosotros have been solving quadratic equations of the form \(a x^{2}+b x+c=0\). We solved for \(10\) and the results were the solutions to the equation.

We are now looking at quadratic functions of the class \(f(x)=a x^{ii}+b x+c\). The graphs of these functions are parabolas. The \(ten\)-intercepts of the parabolas occur where \(f(x)=0\).

For instance:

Quadratic equation

\(\begin{aligned}x^{ii}-2 ten-15 & =0\quad \text{Permit}\:f(x)=0 \\ (10-five)(x+3) &=0 \\ x-v=0\:\:x+three & =0 \\ x=5\:\:\:10&=-three\end{aligned}\)

Quadratic function

\(\brainstorm{aligned} f(ten) &=x^{2}-2 x-15 \\ 0 &=ten^{2}-2 x-15 \\ 0 &=(ten-5)(x+3) \\ x-5 &=0 \quad 10+iii=0 \\ ten &=5 \quad x=-3 \\(5,0) & \text { and }(-iii,0) \\& 10\text { -intercepts } \end{aligned}\)

The solutions of the quadratic function are the \(x\) values of the \(x\)-intercepts.

Earlier, we saw that quadratic equations accept \(two, i\), or \(0\) solutions. The graphs below show examples of parabolas for these three cases. Since the solutions of the functions give the \(x\)-intercepts of the graphs, the number of \(x\)-intercepts is the same as the number of solutions.

Previously, we used the discriminant to decide the number of solutions of a quadratic role of the form \(a ten^{two}+b x+c=0\). At present we tin can utilise the discriminant to tell us how many \(x\)-intercepts in that location are on the graph.

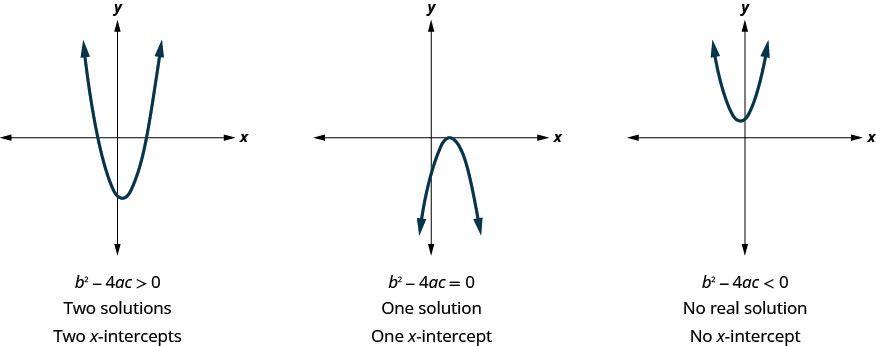
Before you to notice the values of the \(x\)-intercepts, you lot may desire to evaluate the discriminant so yous know how many solutions to expect.

Example \(\PageIndex{5}\)

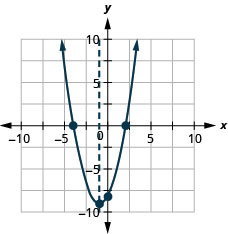
Find the intercepts of the parabola for the function \(f(ten)=5 x^{2}+x+four\).

Solution:

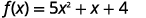To find the \(y\)-intercept, let \(x=0\) and solve for \(f(x)\).
	When \(ten=0\), and then \(f(0)=four\). The \(y\)-intercept is the bespeak \((0,four)\).
To find the \(ten\)-intercept, let \(f(x)=0\) and solve for \(ten\).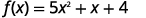
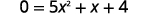Discover the value of the discriminant to predict the number of solutions which is also the number of \(x\)-intercepts.
\(\brainstorm{array}{c}{b^{2}-4 a c} \\ {1^{2}-4 \cdot 5 \cdot iv} \\ {1-80} \\ {-79}\finish{array}\)
	Since the value of the discriminant is negative, at that place is no existent solution to the equation. At that place are no \(x\)-intercepts.

Exercise \(\PageIndex{9}\)

Discover the intercepts of the parabola whose function is \(f(x)=3 x^{two}+4 x+four\).

Answer

\(y\)-intercept: \((0,4)\) no \(x\)-intercept

Exercise \(\PageIndex{10}\)

Find the intercepts of the parabola whose part is \(f(10)=x^{two}-4 x-5\)

Respond

\(y\)-intercept: \((0,-five)\) \(x\)-intercepts \((-1,0),(5,0)\)

Graph Quadratic Functions Using Properties

Now we have all the pieces we demand in order to graph a quadratic part. We simply need to put them together. In the adjacent case we will see how to practice this.

Example \(\PageIndex{6}\) How to Graph a Quadratic Function Using Properties

Graph \(f(x)=x^{two}-6x+8\) by using its backdrop.

Solution:

Step 1: Determine whether the parabola opens upwards or down.	Look \(a\) in the equation \(f(ten)=ten^{2}-6x+8\) Since \(a\) is positive, the parabola opens upwards.	\(f(x)=10^{two}-6x+8\) \(\colour{red}{a=1, b=-6, c=eight}\) The parabola opens upward.
Step two: Detect the axis of symmetry.	\(f(x)=x^{two}-6x+8\) The axis of symmetry is the line \(ten=-\frac{b}{2 a}\).	Axis of Symmetry \(10=-\frac{b}{two a}\) \(x=-\frac{(-6)}{2 \cdot 1}\) \(ten=3\) The axis of symmetry is the line \(10=iii\).
Pace 3: Find the vertex.	The vertex is on the axis of symmetry. Substitute \(x=three\) into the function.	Vertex \(f(ten)=10^{2}-6x+eight\) \(f(3)=(\color{cerise}{iii}\colour{black}{)}^{2}-6(\colour{red}{iii}\color{black}{)}+8\) \(f(iii)=-1\) The vertex is \((three,-1)\).
Stride 4: Find the \(y\)-intercept. Find the point symmetric to the \(y\)-intercept across the centrality of symmetry.	We find \(f(0)\). We use the centrality of symmetry to detect a indicate symmetric to the \(y\)-intercept. The \(y\)-intercept is \(three\) units left of the axis of symmetry, \(x=3\). A point \(3\) units to the right of the centrality of symmetry has \(x=six\).	\(y\)-intercept \(f(x)=x^{2}-6 x+8\) \(f(0)=(\colour{cherry}{0}\color{blackness}{)}^{2}-6(\colour{cherry-red}{0}\color{black}{)}+eight\) \(f(0)=8\) The \(y\)-intercept is \((0,eight)\). Betoken symmetric to \(y\)-intercept: The point is \((6,8)\).
Footstep v: Detect the \(ten\)-intercepts. Notice additional points if needed.	We solve \(f(x)=0\). We can solve this quadratic equation by factoring.	\(x\)-intercepts \(f(x)=x^{ii}-6 x+8\) \(\color{ruby}{0}\color{blackness}{=}x^{ii}-6x+viii\) \(\colour{ruby}{0}\color{blackness}{=}(x-2)(x-iv)\) \(x=ii or x=4\) The \(x\)-intercepts are \((ii,0)\) and \((four,0)\).
Stride 6: Graph the parabola.	Nosotros graph the vertex, intercepts, and the indicate symmetric to the \(y\)-intercept. We connect these \(v\) points to sketch the parabola.

Exercise \(\PageIndex{11}\)

Graph \(f(10)=x^{ii}+2x-8\) past using its backdrop.

Answer

Practice \(\PageIndex{12}\)

Graph \(f(x)=x^{ii}-8x+12\) by using its backdrop.

Answer

We listing the steps to take in order to graph a quadratic function here.

To Graph a Quadratic Function Using Backdrop

1. Determine whether the parabola opens upward or downward.
2. Find the equation of the centrality of symmetry.
3. Detect the vertex.
4. Find the \(y\)-intercept. Find the signal symmetric to the \(y\)-intercept across the axis of symmetry.
5. Notice the \(10\)-intercepts. Find boosted points if needed.
6. Graph the parabola.

Nosotros were able to find the \(10\)-intercepts in the final example by factoring. We find the \(x\)-intercepts in the next example by factoring, too.

Practice \(\PageIndex{13}\)

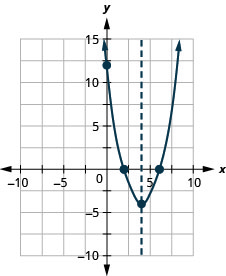
Graph \(f(ten)=3 ten^{two}+12 10-12\) by using its properties.

Answer

Exercise \(\PageIndex{fourteen}\)

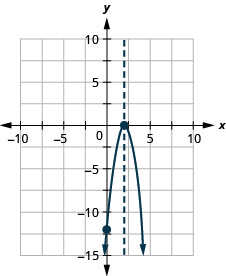
Graph \(f(ten)=iv x^{two}+24 ten+36\) by using its properties.

Answer

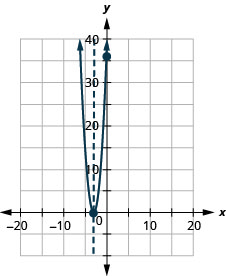
For the graph of \(f(ten)=-x^{2}+6 x-9\), the vertex and the \(x\)-intercept were the aforementioned point. Call up how the discriminant determines the number of solutions of a quadratic equation? The discriminant of the equation \(0=-10^{2}+6x-9\) is \(0\), so there is merely 1 solution. That ways there is only one \(x\)-intercept, and it is the vertex of the parabola.

How many \(x\)-intercepts would you expect to see on the graph of \(f(x)=10^{2}+4 x+v\)?

Practice \(\PageIndex{15}\)

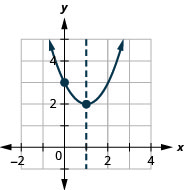
Graph \(f(x)=x^{2}-two x+three\) by using its properties.

Answer

Practise \(\PageIndex{16}\)

Graph \(f(ten)=-3x^{two}-6 x-4\) by using its backdrop.

Answer

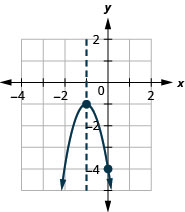
Finding the \(y\)-intercept by finding \(f(0)\) is easy, isn't information technology? Sometimes nosotros need to use the Quadratic Formula to detect the \(x\)-intercepts.

Case \(\PageIndex{nine}\)

Graph \(f(10)=two x^{2}-four x-3\) by using its properties.

Solution:

Since \(a\) is \(two\), the parabola opens upward.
To observe the equation of the axis of symmetry, use \(ten=-\frac{b}{2 a}\).	\(x=-\frac{b}{2 a}\)
	\(ten=-\frac{-4}{2 \cdot 2}\)
	\(x=i\)
	The equation of the axis of symmetry is \(x=1\).
The vertex is on the line \(x=1\).	\(f(x)=2 10^{ii}-4 x-three\)
Detect \(f(ane)\).
	\(f(1)=2-4-three\)
	\(\f(one)=-five)
	The vertex is \((one,-5)\).
The \(y\)-intercept occurs when \(ten=0\).	\(f(x)=two ten^{2}-4 x-3\)
Find \(f(0)\).
Simplify.	\(f(0)=-three\)
	The \(y\)-intercept is \((0,-3)\).
The point \((0,-three)\) is one unit to the left of the line of symmetry.	Betoken symmetric to the \(y\)-intercept is \((2,-iii)\)
The point 1 unit to the correct of the line of symmetry is \((2,3)\).
The \(ten\)-intercept occurs when \(y=0\).	\(f(x)=2 x^{2}-4 ten-3\)
Discover \(f(x)=0\).
Use the Quadratic Formula.	\(10=\frac{-b \pm \sqrt{b^{two}-4 a c}}{2 a}\)
Substitute in the values of \(a,b\) and \(c\).	\(ten=\frac{-(-4) \pm \sqrt{(-iv)^{2}-4(2)(3)}}{2(2)}\)
Simplify.	\(x=\frac{-4 \pm \sqrt{16+24}}{4}\)
Simplify within the radical.	\(x=\frac{4 \pm \sqrt{forty}}{4}\)
Simplify the radical.	\(x=\frac{4 \pm 2 \sqrt{10}}{4}\)
Gene the GCF.	\(x=\frac{2(2 \pm \sqrt{ten})}{4}\)
Remove common factors.	\(x=\frac{two \pm \sqrt{10}}{2}\)
Write as ii equations.	\(x=\frac{2+\sqrt{10}}{2}, \quad x=\frac{2-\sqrt{x}}{two}\)
Approximate the values.	\(x \approx two.5, \quad 10 \approx-0.6\)
	The estimate values of the \(x\)-intercepts are \((2.5,0)\) and \((-0.6,0)\).
Graph the parabola using the points establish.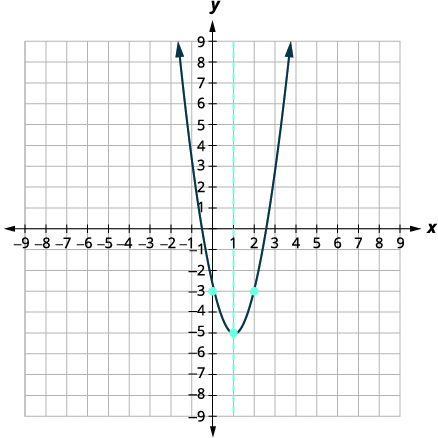

Do \(\PageIndex{17}\)

Graph \(f(x)=5 x^{2}+x x+3\) by using its properties.

Answer

Practise \(\PageIndex{xviii}\)

Graph \(f(x)=-3 ten^{2}-6 x+5\) by using its backdrop.

Answer

Solve Maximum and Minimum Applications

Knowing that the vertex of a parabola is the lowest or highest point of the parabola gives usa an piece of cake way to determine the minimum or maximum value of a quadratic function. The y-coordinate of the vertex is the minimum value of a parabola that opens upwardly. It is the maximum value of a parabola that opens downward. Run into Effigy 9.6.124.

Figure 9.6.124

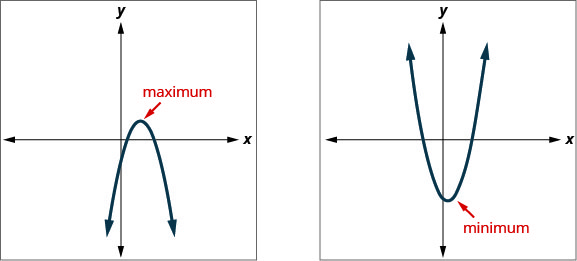
Minimum or Maximum Values of a Quadratic Function

The y-coordinate of the vertex of the graph of a quadratic function is the

• minimum value of the quadratic equation if the parabola opens upward.
• maximum value of the quadratic equation if the parabola opens downward.

Example \(\PageIndex{10}\)

Find the minimum or maximum value of the quadratic part \(f(ten)=ten^{two}+2 x-8\).

Solution:

	\(f(10)=x^{2}+ii ten-eight\)
Since \(a\) is positive, the parabola opens upward. The quadratic equation has a minimum.
Find the equation of the axis of symmetry.	\(x=-\frac{b}{2 a}\)
	\(ten=-\frac{2}{ii \times ane}\)
	\(10=-1\)
	The equation of the axis of symmetry is \(x=-1\).
The vertex is on the line \(ten=-1\).	\(f(x)=x^{two}+2 10-viii\)
Find \(f(-1)\).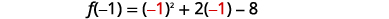
	\(f(-1)=1-2-8\)
	\(f(-1)=-9\)
	The vertex is \((-1,-ix)\).
Since the parabola has a minimum, the \(y\)-coordinate of the vertex is the minimum \(y\)-value of the quadratic equation. The minimum value of the quadratic is \(-nine\) and it occurs when \(x=-ane\).

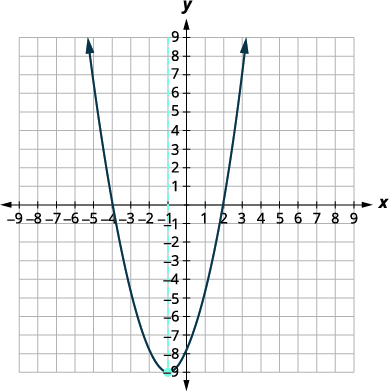Testify the graph to verify the result.

Practice \(\PageIndex{xix}\)

Find the maximum or minimum value of the quadratic office \(f(x)=x^{two}-8 x+12\).

Reply

The minimum value of the quadratic role is \(−4\) and it occurs when \(10=four\).

Exercise \(\PageIndex{20}\)

Find the maximum or minimum value of the quadratic function \(f(x)=-4 x^{2}+xvi x-11\).

Answer

The maximum value of the quadratic function is \(5\) and it occurs when \(x=two\).

Nosotros have used the formula

\(h(t)=-xvi t^{2}+v_{0} t+h_{0}\)

to calculate the height in feet, \(h\), of an object shot up into the air with initial velocity, \(v_{0}\), after \(t\) seconds .

This formula is a quadratic function, so its graph is a parabola. By solving for the coordinates of the vertex \((t,h)\), we can discover how long information technology will take the object to attain its maximum peak. Then we can calculate the maximum superlative.

Case \(\PageIndex{11}\)

The quadratic equation \(h(t)=-16 t^{2}+176 t+4\) models the height of a volleyball hit directly upwards with velocity \(176\) feet per second from a height of \(4\) feet.

1. How many seconds will it take the volleyball to reach its maximum pinnacle?
2. Find the maximum superlative of the volleyball.

Solution:

\(h(t)=-xvi t^{2}+176 t+4\)

Since \(a\) is negative, the parabola opens downwards. The quadratic function has a maximum.

a. Find the equation of the axis of symmetry.

\(\begin{array}{l}{t=-\frac{b}{two a}} \\ {t=-\frac{176}{two(-sixteen)}} \\ {t=5.5}\end{assortment}\)

The equation of the axis of symmetry is \(t=5.v\).

The vertex is on the line \(t=5.five\).

The maximum occurs when \(t=5.5\) seconds.

b. Find \(h(5.5)\).

\(\begin{array}{l}{h(t)=-16 t^{2}+176 t+4} \\ {h(t)=-16(5.five)^{2}+176(5.5)+4}\end{assortment}\)

Use a calculator to simplify.

\(h(t)=488\)

The vertex is \((five.v,488)\).

Since the parabola has a maximum, the \(h\)-coordinate of the vertex is the maximum value of the quadratic function.

The maximum value of the quadratic is \(488\) feet and it occurs when \(t=5.5\) seconds.

Afterward \(5.5\) seconds, the volleyball will reach its maximum top of \(488\) feet.

Practise \(\PageIndex{21}\)

Solve, rounding answers to the nearest tenth.

The quadratic office \(h(t)=-xvi t^{ii}+128 t+32\) is used to find the height of a rock thrown upward from a acme of \(32\) feet at a rate of \(128\) ft/sec. How long will information technology take for the stone to reach its maximum height? What is the maximum height?

Answer

It will take \(4\) seconds for the rock to achieve its maximum meridian of \(288\) feet.

Exercise \(\PageIndex{22}\)

A path of a toy rocket thrown upward from the ground at a charge per unit of \(208\) ft/sec is modeled by the quadratic function of \(h(t)=-16 t^{ii}+208 t\). When will the rocket reach its maximum height? What volition be the maximum superlative?

Answer

It will take \(6.5\) seconds for the rocket to attain its maximum pinnacle of \(676\) feet.

Central Concepts

• Parabola Orientation
  • For the graph of the quadratic part \(f(ten)=a x^{2}+b x+c\), if
    • \(a>0\), the parabola opens up.
    • \(a<0\), the parabola opens downward.
• Axis of Symmetry and Vertex of a Parabola The graph of the function \(f(10)=a ten^{two}+b x+c\) is a parabola where:
  • the axis of symmetry is the vertical line \(x=-\frac{b}{2 a}\).
  • the vertex is a point on the centrality of symmetry, and so its \(x\)-coordinate is \(-\frac{b}{two a}\).
  • the \(y\)-coordinate of the vertex is constitute by substituting \(10=-\frac{b}{2 a}\) into the quadratic equation.
• Discover the Intercepts of a Parabola
  • To observe the intercepts of a parabola whose office is \(f(x)=a x^{ii}+b x+c\):
    • \(y\)-intercept
      • Let \(x=0\) and solve for \(f(10)\).
    • \(x\)-intercepts
      • Let \(f(x)=0\) and solve for \(10\).
• How to graph a quadratic office using properties.
  1. Determine whether the parabola opens upward or down.
  2. Find the equation of the axis of symmetry.
  3. Find the vertex.
  4. Discover the \(y\)-intercept. Detect the point symmetric to the y-intercept beyond the centrality of symmetry.
  5. Find the \(x\)-intercepts. Find additional points if needed.
  6. Graph the parabola.
• Minimum or Maximum Values of a Quadratic Equation
  • The \(y\)-coordinate of the vertex of the graph of a quadratic equation is the
  • minimum value of the quadratic equation if the parabola opens upward.
  • maximum value of the quadratic equation if the parabola opens downward.

Glossary

quadratic function

A quadratic role, where \(a, b\), and \(c\) are existent numbers and \(a≠0\), is a function of the form \(f(x)=ax^{2}+bx+c\).

powellthisings.blogspot.com

Source: https://math.libretexts.org/Bookshelves/Algebra/Intermediate_Algebra_(OpenStax)/09%3A_Quadratic_Equations_and_Functions/907%3A_Graph_Quadratic_Functions_Using_Properties

Share this post